Summary of quadrilaterals

Solved exercise of quadrilaterals

Summary

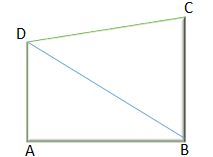
As the word ‘Quad’ means four, and lateral means sides.A quadrilateral is a plane figure that has four sides or edges, and also have four corners or vertices.AC and BD are the diagonals of the quadrilateral ABCD.

Properties of quadrilaterals

Angle sum property of a quadrilateral

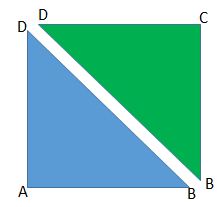
Statement: sum of angles of quadrilateral  is 360 degrees

∴ Sum of the four angles of the quadrilateral is

∴ ∠A + ∠B + ∠C + ∠D = 360°

Types of Quadrilaterals

There are many types of quadrilaterals. quadrilateral have four sides, and the sum of angles of these shapes is 360 degrees.

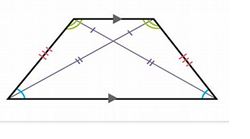
1.Trapezium

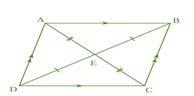
2.Parallelogram

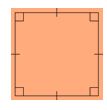
3.Squares

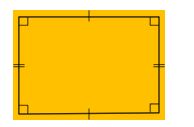
4.Rectangle

5.Rhombus

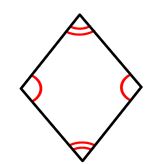
6.Kite

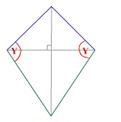
Trapezium

A trapezium is a quadrilateral with one pair of opposite parallel sides.

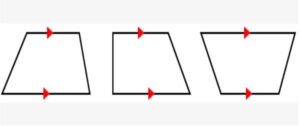
Properties: The parallel sides are called bases. The other two non-parallel sides are called legs. •If the two non-parallel sides are equal and form equal angles at one of the bases, the trapezium is an isosceles trapezium.

Isosceles Trapezoid

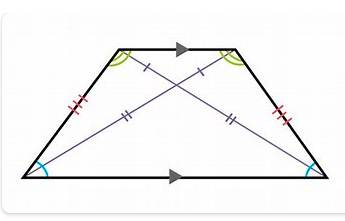
• One Pair of parallel sides (AB and DC)
• One pair of congruent legs (DA and CB)
• Base angles are congruent (M<D=M<C)
• Diagonals are congruent (AC and BD)
• Opposite angles are supplementary (their sum is 180 degree)

Kite

A kite is a quadrilateral that has 2 pairs of equal-length sides and these sides are adjacent to each other.

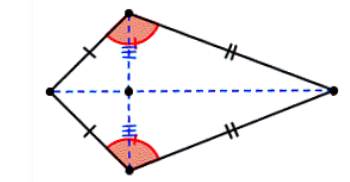
Properties: The two angles are equal where the unequal sides meet. •It can be viewed as a pair of congruent triangles with a common base. •It has 2 diagonals that intersect each other at right angles. •The longer or main diagonal bisects the other diagonal. •A kite is symmetrical about its main diagonal. •The shorter diagonal divides the kite into 2 isosceles triangles.

Parallelogram

A parallelogram is a quadrilateral with two pairs of parallel sides.

PERIMETER OF PARALLELOGRAM: Perimeter of a parallelogram. Is equal to perimeter of a rectangle= 2( l + b ).

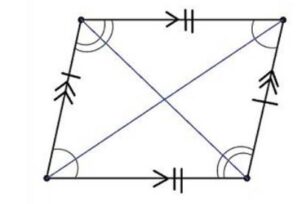
•Opposite sides are parallel and equal in length. •Opposite angles are equal in measure. •Adjacent angles sum up to 180 degrees. •It has 2 diagonals that bisect each other. •Each diagonal divides the parallelogram into 2 congruent triangles. •The two diagonals divide the parallelogram into 4 triangles of equal area. •Square, rectangle, and rhombus are special types of a parallelogram.

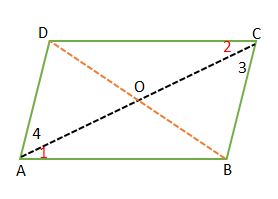
• The opposite side of the parallelogram are of the same length
• The diagonals of a parallelogram bisect each other
• The opposite angles are of equal measure
• The opposite angles are of equal measure
• The sum of two adjacent angles of a parallelogram is equal to 180 degrees

Exercise for class 8

Exercise 4.3

1. Given a parallelogram ABCD. Complete each statement along with the definition or property used.

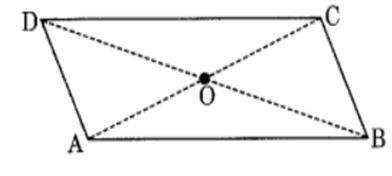
(i) AD = …………
(ii) ∠DCB = ………
(iii) OC = ………
(iv) ∠DAB + ∠CDA = ……..

Solution:

(i) AD = BC[Opposite sides of a parallelogram are equal]

(iii) OC = OA[Diagonals of a parallelogram bisect each other]

(iv) ∠DAB + ∠CDA = 180[Adjacent angles of a parallelogram are supplementary]

2. Consider the following parallelograms. Find the values of the unknowns x, y, z

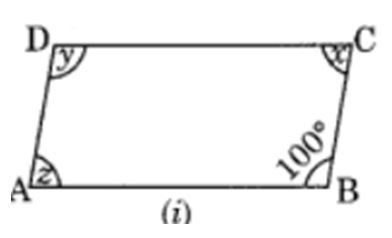
Solution:(i) ABCD is a parallelogram.

∠B = ∠D [Opposite angles of a parallelogram are equal

∠D = 100°

⇒ y = 100°

∠A + ∠B = 180°

 [Adjacent angles of a parallelogram are supplementary]

⇒ z + 100° = 180°

⇒ z = 180° – 100° = 80°

∠A = ∠C[Opposite angles of a ||gm]

x = 80°

Hence x = 80°, y = 100° and z = 80°

(ii) PQRS is a parallelogram

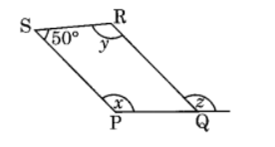
∠P + ∠S = 180 [Adjacent angles of parallelogram]

⇒ x + 50° = 180°

x = 180° – 50° = 130°

Now, ∠P = ∠R[Opposite angles are equal]

⇒ x = y

⇒ y = 130°

Also, y = z [Alternate angles

z = 130°

Hence, x = 130°, y = 130° and z = 130

(iii) ABCD is a rhombus

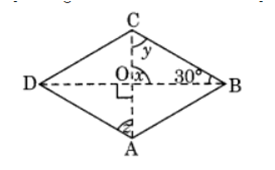
[∵ Diagonals intersect at 90°]

x = 90°

Now in ∆OCB,

x + y + 30° = 180°(Angle sum property)

⇒ 90° + y + 30° = 180°

⇒ y + 120° = 180°

⇒ y = 180° – 120° = 60°

y = z (Alternate angles)

⇒ z = 60°

Hence, x = 90°, y = 60° and z = 60°.

3. Can a quadrilateral ABCD be a parallelogram if

(i) ∠D + ∠B = 180°?
(ii) AB = DC = 8 cm, AD = 4 cm and BC = 4.4 cm?
(iii) ∠A = 70° and ∠C = 65°?

Solution

(i) For ∠D + ∠B = 180, quadrilateral ABCD may be a parallelogram if following conditions are also fulfilled.

(a) The sum of measures of adjacent angles should be 180°.

(b) Opposite angles should also be of same measures. So, ABCD can be but need not be a parallelogram.

(ii) Given: AB = DC = 8 cm, AD = 4 cm, BC = 4.4 cm

In a parallelogram, opposite sides are equal.

Here AD ≠ BC

Thus, ABCD cannot be a parallelogram.

4. Draw a rough figure of a quadrilateral that is not a parallelogram but has exactly two opposite angles of equal measure.

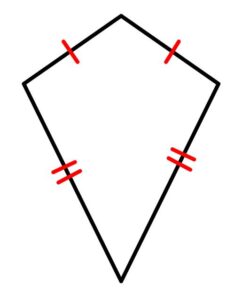
Solution:

ABCD is a rough figure of a quadrilateral in which ∠A = ∠C but it is not a parallelogram.

It is a kite.

5. The measures of two adjacent angles of a parallelogram are in the ratio 3 : 2. Find the measure of each of the angles of the parallelogram.

Solution: Let ABCD is parallelogram such that
∠B : ∠C = 3 : 2

Let ∠B = 3x° and m∠C = 2x°

∠B + ∠C = 180° (Sum of adjacent angles = 180°)

3x + 2x = 180°

⇒ 5x = 180°
⇒ x = 36°

Thus, ∠B = 3 × 36 = 108°

∠C = 2 × 36° = 72°
∠B = ∠D = 108°
and ∠A = ∠C = 72°

Hence, the measures of the angles of the parallelogram are 108°, 72°, 108° and 72°.

7. The adjacent figure HOPE is a parallelogram. Find the angle measures x, y and z. State the properties you use to find them.

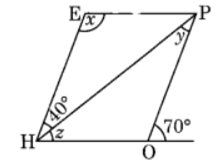
Solution:∠y = 40° (Alternate angles)
∠z + 40° = 70° (Exterior angle property)

⇒ ∠z = 70° – 40° = 30°
z = ∠EPH (Alternate angle)

In ∆EPH
∠x + 40° + ∠z = 180° (Adjacent angles)

⇒ ∠x + 40° + 30° = 180°
⇒ ∠x + 70° = 180°
⇒ ∠x = 180° – 70° = 110°

Hence x = 110°, y = 40° and z = 30°.

8. The following figures GUNS and RUNS are parallelograms. Find x and y. (Lengths are in cm)

i)GU=SN(opposite sides of a parallelogram)

3y-1=26

=3y=26+1

=3y=27

y=27/3=9

similarly, GS=UN

3x=18

x=18/3=6

Hence, x=6cmand y=9cm

(ii) Since, the diagonals of a parallelogram bisect each other

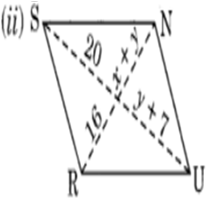
Therefore, OU = OS

⇒ y + 7 = 20

⇒ y = 20 – 7 = 13
Also, ON = OR

⇒ x + y = 16
⇒ x + 13 = 16

x = 16 – 13 = 3
Hence, x = 3 cm and y = 13 cm.

9. In the above figure both RISK and CLUE are parallelograms. Find the value of x.

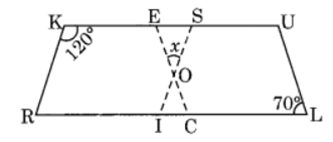
Solution: Here RISK and CLUE are two parallelograms.

∠1 = ∠L = 70° (Opposite angles of a parallelogram)

∠K + ∠2 = 180°
Sum of adjacent angles is 180°

120° + ∠2 = 180°
∠2 = 180° – 120° = 60°

In ∆OES,∠x + ∠1 + ∠2 = 180° (Angle sum property)

⇒ ∠x + 70° + 60° = 180°
⇒ ∠x + 130° = 180°

⇒ ∠x = 180° – 130° = 50°
Hence x = 50°

10. Explain how this figure is a trapezium. Which of its two sides are parallel?

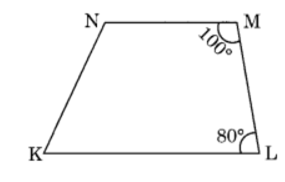
Solution:∠M + ∠L = 100° + 80° = 180°

∠M and ∠L are the adjacent angles, and sum of adjacent interior angles is 180°

KL is parallel to NM
Hence KLMN is a trapezium.

11. Find ∠C in below figure if AB || DC

Solution:Given that AB || DC

∠B + ∠C = 180° (Sum of adjacent angles of a parallelogram is 180°)

120° + ∠C = 180°

∠C = 180° – 120° = 60°
Hence ∠C = 60°

12. Find the measure of ∠P and ∠S if SP || RQ in figure, is there any other method to find ∠P?)

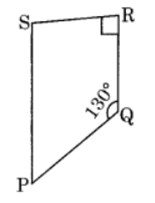
Solution:Given that ∠Q = 130° and ∠R = 90°
SP || RQ (given)

∠P + ∠Q = 180° (Adjacent angles)

⇒ ∠P + 130° = 180°
⇒ ∠P = 180° – 130° = 50°

and, ∠S + ∠R = 180° (Adjacent angles)

⇒ ∠S + 90° = 180°
⇒ ∠S = 180° – 90° = 90°

Alternate Method:

∠Q = 130°, ∠R = 90° and ∠S = 90°

We know that
∠P + ∠Q + ∠R + ∠Q = 360° (Angle sum property of quadrilateral)

⇒ ∠P + 130° + 90° + 90° = 360°
⇒ ∠P + 310° = 360°

⇒ ∠P = 360° – 310° = 50°
Hence ∠P = 50°

Rhombus

A rhombus is a quadrilateral with sides of equal length.

Since the opposite sides of a rhombus have the same length, it is also a parallelogram.

The diagonals of a rhombus are perpendicular bisectors of one another.

Properties of Special Parallelograms

Rectangle: A rectangle is a parallelogram with equal angles and each angle is equal to 90∘.
Properties:

•Opposite sides of a rectangle are parallel and equal.

•The length of diagonals of a rectangle is equal.

All the interior angles of a rectangle are equal to 90∘

•The diagonals of a rectangle bisect each other at the point of intersection.

Square

In a square the diagonals

In a square all the sides are equal

•bisect one another

•are of equal length

•are perpendicular to one another

Example:7

RIce is a rhombus find x,y,z justify

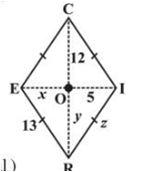
x=OE

=OI(diagonals bisect

=5

y=or

=oc (diagonal bisect

=12

z=side of the rhombu

=13(all sides are equal

Exercise 4.4

1. State whether True or False. Solution:

• a) All rectangles are squares. False
• (b) All rhombuses are parallelograms. True
• (c) All squares are rhombuses and also rectangles. True
• (d) All squares are not parallelograms. False
• (e) All kites are rhombuses False
• (f) All rhombuses are kites. True
• (g) All parallelograms are trapeziums. True
• (h) All squares are trapeziums. True

2. Identify all the quadrilaterals that have
(a) four sides of equal length
(b) four right angles

Solution:(a) Squares and rhombuses.
(b) Rectangles and squares.

3. Explain how a square is
(i) a quadrilateral
(ii) a parallelogram
(iii) a rhombus
(iv) a rectangle

Solution:(i) Square is a quadrilateral because it is closed with four line segments.

(ii) Square is a parallelogram due to the following properties:

(a) Opposite sides are equal and parallel.
(b) Opposite angles are equal.

(iii) Square is a rhombus because its all sides are equal and opposite sides are parallel.

(iv) Square is a rectangle because its opposite sides are equal and has equal diagonal.

5. Name the quadrilaterals whose diagonals

(i) bisect each other
(ii) are perpendicular bisectors of each other
(iii) are equal

Solution:

(i) bisect each other

(i) Parallelogram, rectangle, square and rhombus

(ii) are perpendicular bisectors of each other

(ii) Square and rhombus

(iii) are equal

(iii) Rectangle and square

5. Explain why a rectangle is a convex quadrilateral.

Solution:In a rectangle, both of its diagonal lie in its interior. Hence, it is a convex quadrilateral.

6. ABC is a right-angled triangle and O is the midpoint of the side opposite to the right angle. Explain why O is equidistant from A, B and C. (The dotted lines are drawn additionally to help you).

Solution:

Since the right-angled triangle ABC makes a rectangle ABCD by the dotted lines

therefore OA = OB = OC = OD [Diagonals of a rectangle are equal and bisect each other]
Hence, O is equidistant from A, B and C.