Triangles and their Types
9th class mathematics
Triangle
Triangle is a closed curve made up of three line segments. It has three vertices, sides and angles.
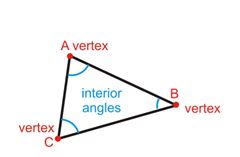
Here, in ∆ABC, •AB, BC and CA are the three sides. •A, B and C are three vertices. •∠A, ∠B and ∠C are the three angles
Types of Triangle
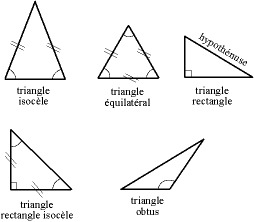
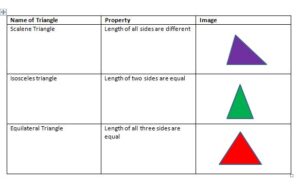
1. There are three types of triangles on the basis of the length of the sides.
2. There are three types of triangles on the basis of angles

Properties of triangles
1. MEDIANS OF A TRIANGLE
Median is the line segment which made by joining any vertex of the triangle with the midpoint of its opposite side. Median divides the side into two equal parts.
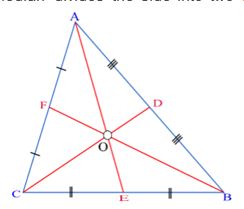
Every triangle has three medians like AE, CD and BF in the above triangle.
The point where all the three medians intersect each other is called Centroid
2. Altitudes of a Triangle
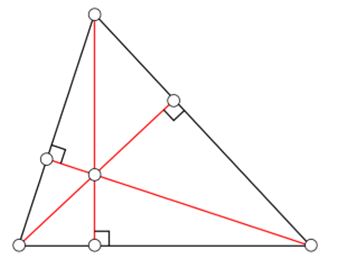
Altitude is the line segment made by joining the vertex and the perpendicular to the opposite side. Altitude is the height if we take the opposite side as the base.
The altitude form angle of 90°.
There are three altitudes possible in a triangle.
The point of intersection of all the three altitudes is called Orthocenter.
The Exterior Angle of a Triangle
If we extend any side of the triangle then we get an exterior angle.
An exterior angle must form a linear pair with one of the interior angles of the triangle.
There are only two exterior angles possible at each of the vertices
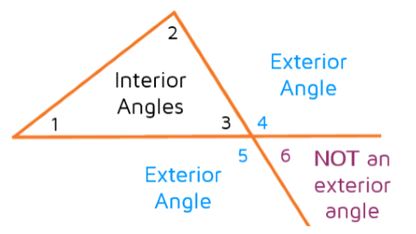
Here ∠4 and ∠5 are the exterior angles of the vertex but ∠6 is not the exterior angle as it is not adjacent to any of the interior angles of the triangle.
3. Exterior Angle Property of the Triangle
An Exterior angle of a triangle will always be equal to the sum of the two opposite interior angles of the triangle.
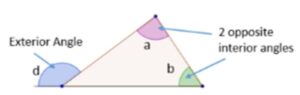
Here, ∠d = ∠a + ∠b
This is called the Exterior angle property of a triangle.
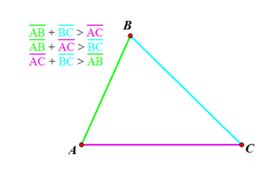
Sum of the length of the two sides of a triangle
Sum of the length of the two sides of a triangle will always be greater than the third side, whether it is an equilateral, isosceles or scalene triangle.
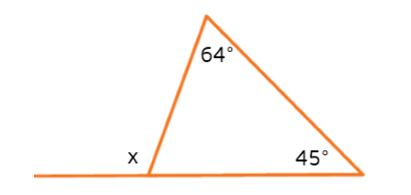
Example
Find the value of “x”.
X is the exterior angle of the triangle and the two given angles are the opposite interior angles.
Hence,
x = 64°+ 45°
x = 109°
4. Angle Sum Property of a Triangle
This property says that the sum of all the interior angles of a triangle is 180°.
Two Special Triangles
1. Equilateral Triangle
It is a triangle in which all the three sides and angles are equal.
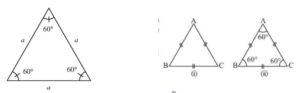
- all sides have same length
- II)each angle hs measure 600
2. Isosceles Triangle
It is a triangle in which two sides are equal and the base angles opposite to the equal sides are also equal.
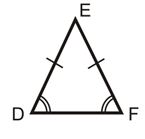
i)two sides have same length
ii)base angle opposite to the equal sides are equal.
3. Right Angled Triangle
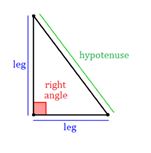
A right-angled triangle is a triangle which has one of its angles as 90° and the side opposite to that angle is the largest leg of the triangle which is known as Hypotenuse .the other two sides are called Legs.
Pythagoras theorem
In a right angle triangle
Hypotenuse)2 = (base)2 + (height)2
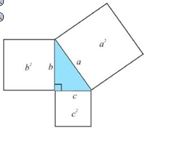
The reverse of Pythagoras theorem is also applicable, i.e. if the Pythagoras property holds in a triangle then it must be a right-angled triangle.
Sum of the length of the two sides of a triangle
Sum of the length of the two sides of a triangle will always be greater than the third side, whether it is an equilateral, isosceles or scalene triangle.
Solved exercises of Triangles and its Types
9th std Exercise 5.1
1. In quadrilateral ACBD, AC = AD and AB bisects ∠ A (see figure). Show that ∆ABC ≅ ∆ABD. What can you say about BC and BD?
Solution:
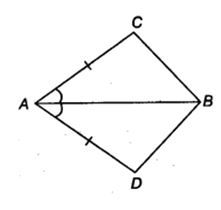
In quadrilateral ACBD, we have AC = AD and AB being the bisector of ∠A.
Now, In ∆ABC and ∆ABD,
AC = AD (Given)
∠ CAB = ∠ DAB ( AB bisects ∠ CAB)
and AB = AB (Common)
∴ ∆ ABC ≅ ∆ABD (By SAS congruence axiom)
∴ BC = BD (By CPCT)
2. ABCD is a quadrilateral in which AD = BC and ∠ DAB = ∠ CBA (see figure). Prove that
(i) ∆ABD ≅ ∆BAC
(ii) BD = AC
(iii) ∠ABD = ∠ BAC
Solution
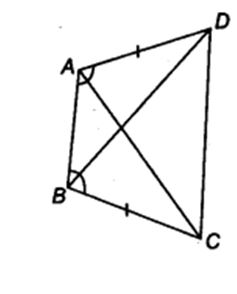
In quadrilateral ACBD, we have AD = BC and ∠ DAB = ∠ CBA
(i)In ∆ ABC and ∆ BAC,
AD = BC (Given)
∠DAB = ∠CBA (Given)
AB = AB (Common)
∴ ∆ ABD ≅ ∆BAC (By SAS congruence).
(ii)Since ∆ABD ≅ ∆BAC
⇒ BD = AC [By C.P.C.T.]
(iii) Since ∆ABD ≅ ∆BAC
⇒ ∠ABD = ∠BAC [By C.P.C.T.].
3. AD and BC are equal perpendiculars to a line segment AB (see figure). Show that CD bisects AB.
Solution

In ∆BOC and ∆AOD, we have
∠BOC = ∠AOD
BC = AD [Given]
∠BOC = ∠AOD [Vertically opposite angles]
∴ ∆OBC ≅ ∆OAD [By AAS congruency]
⇒ OB = OA [By C.P.C.T.]
i.e., O is the mid-point of AB.
Thus, CD bisects AB.
4. l and m are two parallel lines intersected by another pair of parallel lines p and q (see figure). Show that ∆ABC = ∆CDA.
Solution
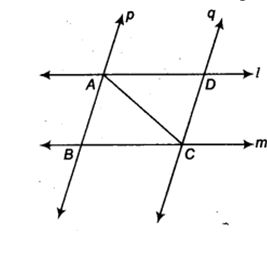
∵ p || q and AC is a transversal,
∴ ∠BAC = ∠DCA …(1) [Alternate interior angles]
Also l || m and AC is a transversal,
∴ ∠BCA = ∠DAC …(2)
[Alternate interior angles]
Now, in ∆ABC and ∆CDA, we have
∠BAC = ∠DCA [From (1)]
CA = AC [Common]
∠BCA = ∠DAC [From (2)]
∴ ∆ABC ≅ ∆CDA [By ASA congruency]
5. Line l is the bisector of an ∠ A and ∠ B is any point on l. BP and BQ are perpendiculars from B to the arms of LA (see figure). Show that
i) ∆APB ≅ ∆AQB
(ii) BP = BQ or B is equidistant from the arms ot ∠A.
Solution:
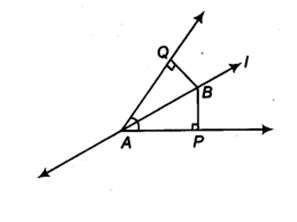
We have, l is the bisector of ∠QAP.
∴ ∠QAB = ∠PAB
∠Q = ∠P [Each 90°]
∠ABQ = ∠ABP [By angle sum property of A]
Now, in ∆APB and ∆AQB, we have
∠ABP = ∠ABQ [Proved above]
AB = BA [Common]
∠PAB = ∠QAB [Given]
∴ ∆APB ≅ ∆AQB [By ASA congruency]
Since ∆APB ≅ ∆AQB
⇒ BP = BQ [By C.P.C.T.]
i. e., [Perpendicular distance of B from AP]
= [Perpendicular distance of B from AQ]
Thus, the point B is equidistant from the arms of ∠A.
6. In figure, AC = AE, AB = AD and ∠BAD = ∠EAC. Show that BC = DE.
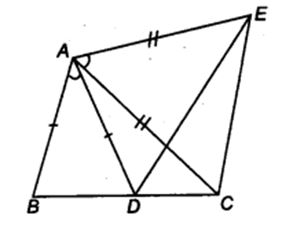
Solution:
We have, ∠BAD = ∠EAC
Adding ∠DAC on both sides, we have
∠BAD + ∠DAC = ∠EAC + ∠DAC
⇒ ∠BAC = ∠DAE
Now, in ∆ABC and ∆ADE. we have
∠BAC = ∠DAE [Proved above]
AB = AD [Given]
AC = AE [Given]
∴ ∆ABC ≅ ∆ADE [By SAS congruency]
⇒ BC = DE [By C.P.C.T.]
7. AS is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠ BAD = ∠ ABE and ∠ EPA = ∠ DPB. (see figure). Show that
(i) ∆DAP ≅ ∆EBP
(ii) AD = BE
Solution:
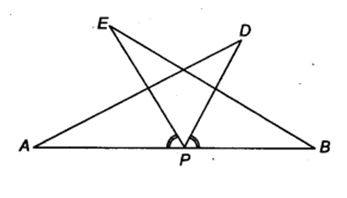
We have, P is the mid-point of AB.
∴ AP = BP
∠EPA = ∠DPB [Given]
Adding ∠EPD on both sides, we get
∠EPA + ∠EPD = ∠DPB + ∠EPD
⇒ ∠APD = ∠BPE
(i) Now, in ∆DAP and ∆EBP, we have
∠PAD = ∠PBE [ ∵∠BAD = ∠ABE]
AP = BP [Proved above]
∠DPA = ∠EPB [Proved above]
∴ ∆DAP ≅ ∆EBP [By ASA congruency]
ii) Since, ∆ DAP ≅ ∆ EBP
⇒ AD = BE [By C.P.C.T.]
8.In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see figure). Show that
i) ∆AMC ≅ ∆BMD
(ii) ∠DBC is a right angle
(iii) ∆DBC ≅ ∆ACB
iv) CM = 1/2 AB
Solution
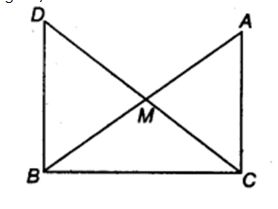
Since M is the mid – point of AB.
∴ BM = AM
(i) In ∆AMC and ∆BMD, we have
CM = DM [Given]
∠AMC = ∠BMD [Vertically opposite angles]
AM = BM [Proved above]
∴ ∆AMC ≅ ∆BMD [By SAS congruency]
(ii) Since ∆AMC ≅ ∆BMD
⇒ ∠MAC = ∠MBD [By C.P.C.T.]
But they form a pair of alternate interior angles.
∴ AC || DB
Now, BC is a transversal which intersects parallel lines AC and DB,
∴ ∠BCA + ∠DBC = 180° [Co-interior angles]
But ∠BCA = 90° [∆ABC is right angled at C]
∴ 90° + ∠DBC = 180°
⇒ ∠DBC = 90°
(iii) Again, ∆AMC ≅ ∆BMD [Proved above]
∴ AC = BD [By C.P.C.T.]
Now, in ∆DBC and ∆ACB, we have
BD = CA [Proved above]
∠DBC = ∠ACB [Each 90°]
BC = CB [Common]
∴ ∆DBC ≅ ∆ACB [By SAS congruency]
(iv) As ∆DBC ≅ ∆ACB
DC = AB [By C.P.C.T.]
But DM = CM [Given]
∴ CM = 1/2 DC = 1/2 AB
⇒ CM = 1/2 AB
class 9 Exercise 5.2
1.In an isosceles triangle ABC, with AB = AC, the bisectors of ∠B and ∠C intersect each other at 0. Join A to 0. Show that
(i) OB = OC
(ii) AO bisects ∠A
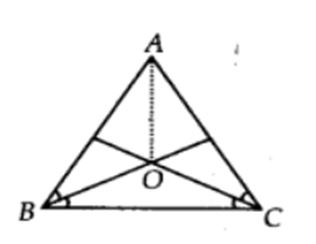
Solution:
i) in ∆ABC, we have
AB = AC [Given]
∴ ∠ABC = ∠ACB [Angles opposite to equal sides are equal]
BO And CO are the bisector of ∠ABC and ∠ACB
Hence ∠OBC = ∠OCB
⇒ OC = OB [Sides opposite to equal angles of a ∆ are equal].
∠OBC = ∠OCB
ii0From ∆ AOB and ∆AOC
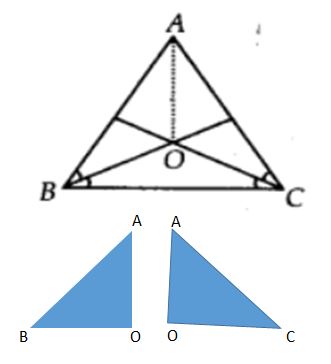
⇒ OC = OB [Sides opposite to equal angles of a ∆ are equal]
∠OBA = ∠OCA
OB = OC [Proved above]
∆ABO ≅ ∆ACO [By SAS congruency]
⇒ ∠OAB = ∠OAC [By C.P.C.T.]
⇒ AO bisects ∠A.
2. ABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively (see figure). Show that these altitudes are equal.
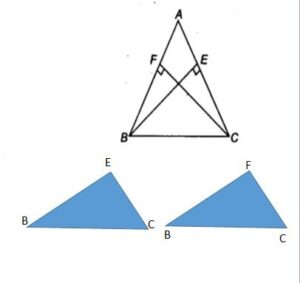
Solution:
∆ABC is an isosceles triangle.
∴ AB = AC
⇒ ∠ACB = ∠ABC [Angles opposite to equal sides of a Aare equal]
⇒ ∠BCE = ∠CBF
Now, in ∆BEC and ∆CFB
∠BCE = ∠CBF [Proved above]
∠BEC = ∠CFB [Each 90°]
BC = CB [Common]
∴ ∆BEC ≅ ∆CFB [By AAS congruency]
So, BE = CF [By C.P.C.T.]
3. ABC and DBC are isosceles triangles on the same base BC (see figure). Show that ∠ ABD = ∠ACD.
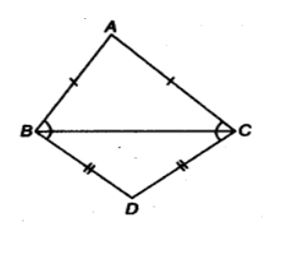
Solution:
In ∆ABC, we have
AB = AC [ABC is an isosceles triangle]
∴ ∠ABC = ∠ACB …(1)
[Angles opposite to equal sides of a ∆ are equal]
Again, in ∆BDC, we have
BD = CD [BDC is an isosceles triangle]
∴ ∠CBD = ∠BCD …(2)[Angles opposite to equal sides of a A are equal
Adding (1) and (2), we have
∠ABC + ∠CBD = ∠ACB + ∠BCD
⇒ ∠ABD = ∠ACD.
4. ∆ABC is an isosceles triangle in which AB = AC. Side BA is produced to D such that AD = AB (see figure). Show that ∠BCD is a right angle.
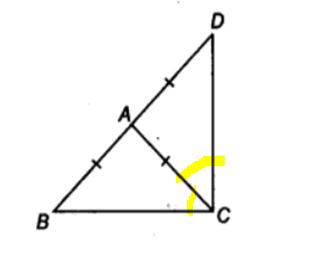
Solution:
AB = AC [Given] …(1)
AB = AD [Given] …(2)
From (1) and (2), we have
AC = AD
[∠ABC = ∠ACB (Angles opposite to equal sides of a A are equal)]
From ∆ ABC,
∠ABC + ∠ACB + ∠BAC = 180[∠ABC = ∠ACB (Angles opposite to equal sides of a A are equal)]
∠ACB + ∠ACB + ∠BAC = 180
⇒ 2∠ACB + ∠BAC = 180° …(3)
Similarly, in ∆ACD,
∠ADC + ∠ACD + ∠CAD = 180°[∠ADC = ∠ACD (Angles opposite to equal sides of a A are equal)]
⇒ 2∠ACD + ∠CAD = 180° …(4)
Adding (3) and (4), we have
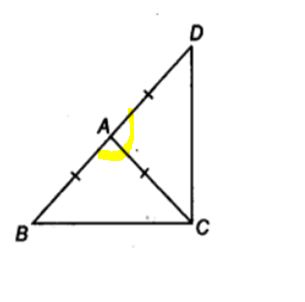
2∠ACB + ∠BAC + 2 ∠ACD + ∠CAD = 180° +180°
⇒ 2[∠ACB + ∠ACD] + [∠BAC + ∠CAD] = 360°
⇒ 2∠BCD +180° = 360° [∠BAC and ∠CAD form a linear pair]
⇒ 2∠BCD = 360° – 180° = 180°
∠BCD = 180 /2
Thus, ∠BCD = 90°
5. ABC is a right angled triangle in which ∠A = 90° and AB = AC, find ∠B and ∠C.
Solution:
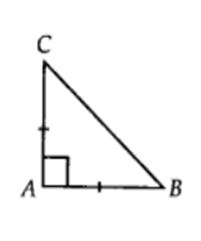
In ∆ABC, we have AB = AC [Given]
∴ Their opposite angles are equal.
⇒ ∠ACB = ∠ABC
Now, ∠A + ∠B + ∠C = 180° [Angle sum property of a ∆]
⇒ 90° + ∠B + ∠C = 180° [∠A = 90°(Given)]
⇒ ∠B + ∠C= 180°- 90° = 90°
But ∠B = ∠C
∠B = ∠C = 90°/2
Thus, ∠B = 45° and ∠C = 45°
6. Show that the angles of an equilateral triangle are 60° each
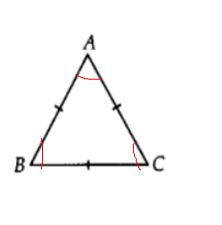
Solution
In ∆ABC, we have
AB = BC = CA
[ABC is an equilateral triangle]
AB = BC
⇒ ∠A = ∠C …(1) [Angles opposite to equal sides of a A are equal]
Similarly, AC = BC
⇒ ∠A = ∠B …(2)
From (1) and (2), we have
∠A = ∠B = ∠C = x (say)
Since, ∠A + ∠B + ∠C = 180° [Angle sum property of a A]
∴ x + x + x = 180o
⇒ 3x = 180°
⇒ x = 60°
∴ ∠A = ∠B = ∠C = 60°
Thus, the angles of an equilateral triangle are 60° each.
Exercise 7.3
1. ∆ABC and ∆DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see figure). If AD is extended to intersect BC at P, show that
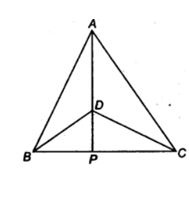
(i) ∆ABD ≅ ∆ACD
(ii) ∆ABP ≅ ∆ACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC.
Solution:
(i) In ∆ABD and ∆ACD, we have
AB = AC [Given]
AD = DA [Common]
BD = CD [Given]
∴ ∆ABD ≅ ∆ACD [By SSS congruency]
∠BAD = ∠CAD [By C.P.C.T.] …(1)
iii) Since, ∆ABP ≅ ∆ACP
⇒ ∠BAP = ∠CAP [By C.P.C.T.]
∴ AP is the bisector of ∠A.
in ∆BDP and ∆CDP,
we have BD = CD [Given]
DP = PD [Common]
BP = CP [ ∵ ∆ABP ≅ ∆ACP]
⇒ A BDP = ACDP [By SSS congruency]
∴ ∠BDP = ∠CDP [By C.P.C.T.]
⇒ DP (or AP) is the bisector of ∠BDC
∴ AP is the bisector of ∠A as well as ∠D.
(iv) As, ∆ABP ≅ ∆ACP
⇒ ∠APS = ∠APC, BP = CP [By C.P.C.T.]
But ∠APB + ∠APC = 180° [Linear Pair]
∴ ∠APB = ∠APC = 90°
⇒ AP ⊥ BC, also BP = CP
Hence, AP is the perpendicular bisector of BC.
Exercise 5.3
1. ∆ABC and ∆DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see figure). If AD is extended to intersect BC at P, show that
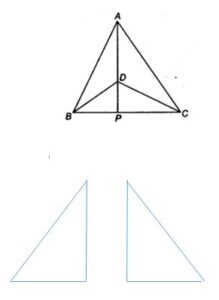
(i) ∆ABD ≅ ∆ACD
(ii) ∆ABP ≅ ∆ACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC.
Solution:(i) In ∆ABD and ∆ACD, we have
AB = AC [Given]
AD = DA [Common]∴ ∆ABD ≅ ∆ACD [By SSS congruency]
∠BAD = ∠CAD [By C.P.C.T.] …(1)
BD = CD [Given]
∴ ∆ABD ≅ ∆ACD [By SSS congruency]
∠BAD = ∠CAD [By C.P.C.T.] …(1)
(iii) Since, ∆ABP ≅ ∆ACP
⇒ ∠BAP = ∠CAP [By C.P.C.T.]
∴ AP is the bisector of ∠A.
in ∆BDP and ∆CDP,
we have BD = CD [Given]
DP = PD [Common]
BP = CP [ ∵ ∆ABP ≅ ∆ACP]
⇒ A BDP = ACDP [By SSS congruency]
∴ ∠BDP = ∠CDP [By C.P.C.T.]
⇒ DP (or AP) is the bisector of ∠BDC
∴ AP is the bisector of ∠A as well as ∠D.
(iv) As, ∆ABP ≅ ∆ACP
⇒ ∠APS = ∠APC, BP = CP [By C.P.C.T.]
But ∠APB + ∠APC = 180° [Linear Pair]
∴ ∠APB = ∠APC = 90°
⇒ AP ⊥ BC, also BP = CP
Hence, AP is the perpendicular bisector of BC.
2. AD is an altitude of an isosceles triangle ABC in which AB = AC. Show that
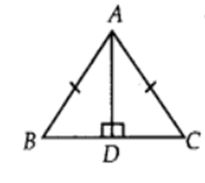
(i) AD bisects BC
(ii) AD bisects ∠A
Solution
ADB = ∠ADC [Each 90°]
AD = DA [Common]
∴ ∆ABD ≅ ∆ACD [By RHS congruency]
So, BD = CD [By C.P.C.T.]
⇒ D is the mid-point of BC or AD bisects BC.
(ii) Since, ∆ABD ≅ ∆ACD,
⇒ ∠BAD = ∠CAD [By C.P.C.T.]
So, AD bisects ∠A
3. Two sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and OR and median PN of ∆PQR (see figure). Show that
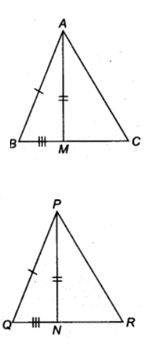
(i) ∆ABC ≅ ∆PQR
(ii) ∆ABM ≅ ∆PQN
Solution
In ∆ABC, AM is the median.
∴ BM = BC/2 , BM = MC ……(1)
In ∆PQR, PN is the median.
∴ QN = QR /2 , QN = NR …(2)
And BC = QR [Given]
⇒ BM = QN …(3) [From (1) and (2)]
(i) In ∆ABM and ∆PQN, we have
AB = PQ , [Given]
AM = PN [Given]
BM = QN [From (3)]
∴ ∆ABM ≅ ∆PQN [By SSS congruency]
(ii) Since ∆ABM ≅ ∆PQN
⇒ ∠B = ∠Q …(4) [By C.P.C.T.]
Now, in ∆ABC and ∆PQR, we have
∠B = ∠Q [From (4)]
AB = PQ [Given]
BC = QR [Given]
∴ ∆ABC ≅ ∆PQR [By SAS congruency]
4. BE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles.
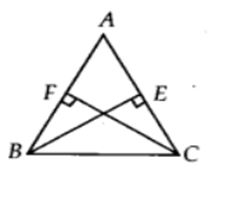
Solution:Since BE ⊥ AC [Given]
∴ BEC is a right triangle such that ∠BEC = 90°
Similarly, ∠CFB = 90°
Now, in right ∆BEC and ∆CFB, we have
BE = CF [Given]
BC = CB [Common hypotenuse]
∠BEC = ∠CFB [Each 90°]
∴ ∆BEC ≅ ∆CFB [By RHS congruency]
So, ∠BCE = ∠CBF [By C.P.C.T.]
∠BCA = ∠CBA
Now, in ∆ABC, ∠BCA = ∠CBA
⇒ AB = AC [Sides opposite to equal angles of a ∆ are equal]
∴ ABC is an isosceles triangle.